Interference of Waves

What is a destructive interference?

Define constructive interference.

What are the types of interference and draw the diagrams to explain the constructive interference.

The maximum intensity of fringes in Young's experiment is $I$. If one of the slit is closed then, the intensity at that place becomes $I_o$. Which of the following relations is true? 

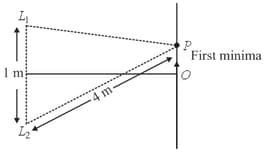
Two loudspeakers $1.0 \mathrm{m}$ apart are coherent sources which emit sound waves of wavelength $1.0 \mathrm{m}$. A microphone moving along OP records the first minimum at $\mathrm{P}$. If ${\mathrm{L}}_2\mathrm{P}$ is $4 \mathrm{m}, {\mathrm{L}}_1\mathrm{P}$ is equal to :

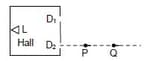
If two coherent sources are placed at a distance $3 \lambda$ from each other symmetric to the centre of the circle shown in the figure, then number of fringes shown on the screen placed along the circumference is :-

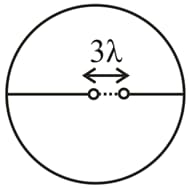
A loudspeaker $L$ is placed in the hall with two doors $D_1$ and $D_2$ as shown below. The distance between $D_1$ and $D_{\mathit{2}}$ is $8.5 \mathrm{m}$. The loudspeaker $L$ is equidistant from $D_{\mathit{1}}$ and $D_{\mathit{2}}$ Monotonic sound waves are emitted from the loudspeaker, and it is found that at point $P$ which is $6.0 \mathrm{m}$ from $D_{\mathit{1}}$ and at point $Q$ the sound intensities are minimum. The line joining $D_{\mathit{1}}\mathit{,}P$ and $Q$ is perpendicular to the line joining $D_{\mathit{1}}$ and $D_{\mathit{2}}$. No other minimum intensity locations can be found between $P \mathrm{and} Q$ and beyond $Q$ along the $PQ$ line. What is the frequency of the sound wave generated by the loudspeaker ?(Speed of sound is $340 \mathrm{m}/\mathrm{s}$ )

The superposing waves are represented by the following equations

$y_1=5 \sin 2\mathrm{\pi }(10t-0.1x)$
$y_2=10 \sin 2\mathrm{\pi }(10t-0.1x)$

Then what would be the ratio of maximum to minimum intensity, $\frac{I_{\max }}{I_{\min }}$?

What is the reason behind the disturbing of incoming and reflected signals when a low flying aircraft passes overhead, which results in slight shaking of the picture on our TV screen?

In Young's double slit experiment, the intensity of light coming from one of the slits is double the intensity from the other slit. The ratio of the maximum intensity to the minimum intensity in the interference fringe pattern observed is

Two waves are described by the equations:

${\mathrm{y}}_1=\mathrm{A}\cos \left(0.5\mathrm{ }\mathrm{\pi }\mathrm{x}-100\mathrm{\pi }\mathrm{t}\right)$

And ${\mathrm{y}}_2=\mathrm{A}\cos \left(0.46\mathrm{ }\mathrm{\pi }\mathrm{x}-92\mathrm{\pi }\mathrm{t}\right)$

Here x and y are in m and t is in s.



The number of maximum heard in one second will be

Intensity and phase of three sound wave reaching at some point in space is ${\mathrm{I}}_0,\mathrm{ }4\mathrm{ }{\mathrm{I}}_0,\mathrm{ }{\mathrm{I}}_0$ and ${10}^{\mathrm{o}},\mathrm{ }{130}^{\mathrm{o}}$ and ${250}^{\mathrm{o}}$ respectively. Resultant intensity at that point will be -

Assertion: Interference obeys the law of conservation of energy.

Reason: The energy is redistributed in case of interference.

A string of length 0.4m and mass ${10}^{-2}$ kg is tightly clamped at the ends. The tension in the string is 1.6 N. Identical wave pulses are produced at one end at equal intervals of time$∆t$. The minimum value of $∆t$, which allows constructive interference between successive pulses is

Two waves of wavelength $99 \mathrm{cm}$ and $100 \mathrm{cm}$ both travelling with velocity $396 \mathrm{m} {\mathrm{s}}^{-1}$ are made to interfere. The number of beats produced by them per second is

Two periodic waves of intensities $I_1$ and $I_2$pass through a region at the same time in the same direction. The sum of the maximum and minimum intensities is